Details of Bending Stresses in beam

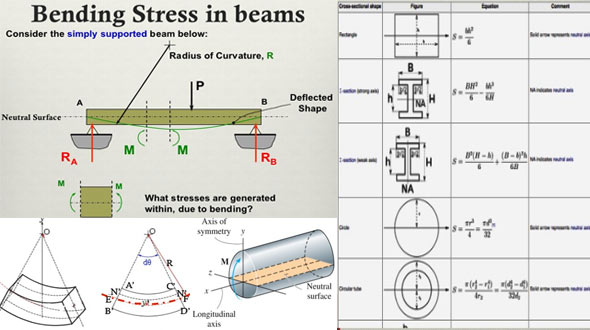
Bending moment creates bending strains on a beam and as a result compressive and tensile stresses are generated. Under positive moment (as generally the case), compressive stresses are formed in the top of the beam and tensile stresses are formed in the bottom.

Bending members should withstand both compressive and tensile stresses.

For induction to bending stress, the rectangular beam and stress diagram are taken into consideration.

When the beam is dependent on some bending moment that stress at any point may be measured with the normal flexure formula : fb = Mc/I

It should be kept in mind that the above expression can only be used when the maximum calculated stress in the beam is under the elastic limit.

The above expression is given on the basis of the supposition that the stress is proportional to the strain and a plane section prior to bending remains plane once the bending is completed.

The value of I/c is a constant for specific section and is defined as the section modulus S.

The flexure formula is provided as follows :-

σ = M/S

Stress fluctuates linearly from the neutral axis to extreme fibers.

When the moment raises, there exist a linear relationship among the moment and the stress unless the stress attains the yield stress F_y

If the moment raises beyond the yield moment, the outermost fibers that was stressed earlier to their yield point will continue to get the same but will yield.

The method will persist with more and more sections of the beam cross section stressed to the yield point unless finally full plastic distribution is addressed.

The plastic moment means the moment that will create complete plasticity in a member cross section and produce a plastic hinge.

The shape factor of a member cross section is described as the proportion of the plastic moment M_p to yield moment M_y.

The shape factor matches 1.50 for rectangular cross sections and differs from about 1.10 to 1.20 for standard rolled beam sections.